
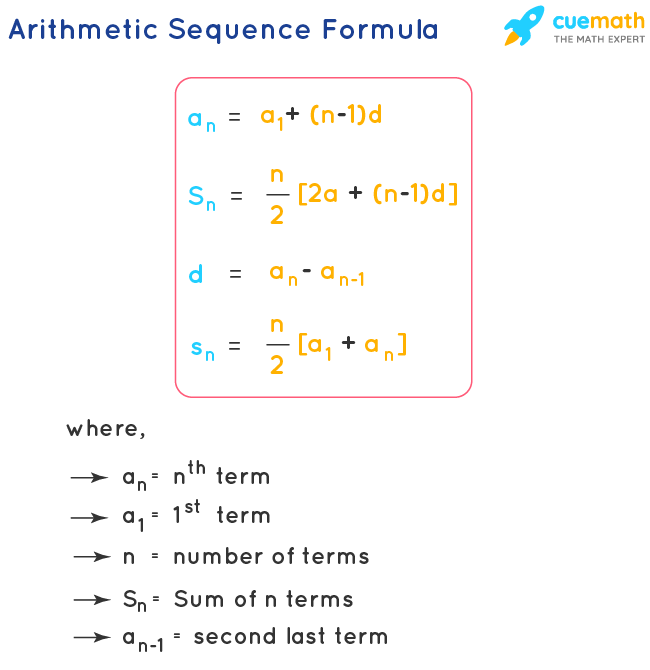
Use this formula to calculate the sum of the first 100 terms of the sequence defined by a n = 2 n − 1. S n = a n + ( a n − d ) + ( a n − 2 d ) + … + a 1Īnd adding these two equations together, the terms involving d add to zero and we obtain n factors of a 1 + a n:Ģ S n = ( a 1 + a n ) + ( a 1 + a n ) + … + ( a n + a 1 ) 2 S n = n ( a 1 + a n )ĭividing both sides by 2 leads us the formula for the nth partial sum of an arithmetic sequence The sum of the first n terms of an arithmetic sequence given by the formula: S n = n ( a 1 + a n ) 2. Therefore, we next develop a formula that can be used to calculate the sum of the first n terms, denoted S n, of any arithmetic sequence. However, consider adding the first 100 positive odd integers. S 5 = Σ n = 1 5 ( 2 n − 1 ) = + + + + = 1 + 3 + 5 + 7 + 9 = 25Īdding 5 positive odd integers, as we have done above, is managable. For example, the sum of the first 5 terms of the sequence defined by a n = 2 n − 1 follows:

is the sum of the terms of an arithmetic sequence.
#ARTMATIC SECENCIES SERIES#
In some cases, the first term of an arithmetic sequence may not be given.Īn arithmetic series The sum of the terms of an arithmetic sequence. Next, use the first term a 1 = − 8 and the common difference d = 3 to find an equation for the nth term of the sequence.Ī n = − 8 + ( n − 1 ) ⋅ 3 = − 8 + 3 n − 3 = − 11 + 3 n Substitute a 1 = − 8 and a 7 = 10 into the above equation and then solve for the common difference d. In this case, we are given the first and seventh term:Ī n = a 1 + ( n − 1 ) d U s e n = 7. In other words, find all arithmetic means between the 1 st and 7 th terms.īegin by finding the common difference d. In fact, any general term that is linear in n defines an arithmetic sequence.įind all terms in between a 1 = − 8 and a 7 = 10 of an arithmetic sequence. In general, given the first term a 1 of an arithmetic sequence and its common difference d, we can write the following:Ī 2 = a 1 + d a 3 = a 2 + d = ( a 1 + d ) + d = a 1 + 2 d a 4 = a 3 + d = ( a 1 + 2 d ) + d = a 1 + 3 d a 5 = a 4 + d = ( a 1 + 3 d ) + d = a 1 + 4 d ⋮įrom this we see that any arithmetic sequence can be written in terms of its first element, common difference, and index as follows:Ī n = a 1 + ( n − 1 ) d A r i t h m e t i c S e q u e n c e Here a 1 = 1 and the difference between any two successive terms is 2. For instance, the sequence 5, 7, 9, 11, 13.
The constant difference is called common difference of that arithmetic progression. For example, the sequence of positive odd integers is an arithmetic sequence, An arithmetic progression or arithmetic sequence ( AP) is a sequence of numbers such that the difference from any succeeding term to its preceding term remains constant throughout the sequence.

Each general term should produce the same sequence, regardless of its starting \(n\)-value.An arithmetic sequence A sequence of numbers where each successive number is the sum of the previous number and some constant d., or arithmetic progression Used when referring to an arithmetic sequence., is a sequence of numbers where each successive number is the sum of the previous number and some constant d.Ī n = a n − 1 + d A r i t h m e t i c S e q u e n c eĪnd because a n − a n − 1 = d, the constant d is called the common difference The constant d that is obtained from subtracting any two successive terms of an arithmetic sequence a n − a n − 1 = d. Find the values of the missing parameters A and B in the following recursive definition of the sequence. So let's say the first term is four, second term is 3 4/5, third term is 3 3/5, fourth term is 3 2/5. Here are the first few terms of the sequence. Write the general term \(a_n\) to describe the number of logs in a row in two different ways. Voiceover g is a function that describes an arithmetic sequence.The stack, as described, has how many rows of logs?.How many seats, in total, are in “A Center” section if there are \(12\) rows in the section?Ĥ) Logs are stacked in a pile with \(48\) logs on the bottom row and \(24\) on the top row.\) and interpret its meaning in words in the context of this problem.


 0 kommentar(er)
0 kommentar(er)
